Energy Changes in Aqueous Solutions (DP IB Chemistry: HL)

Enthalpies of Solution

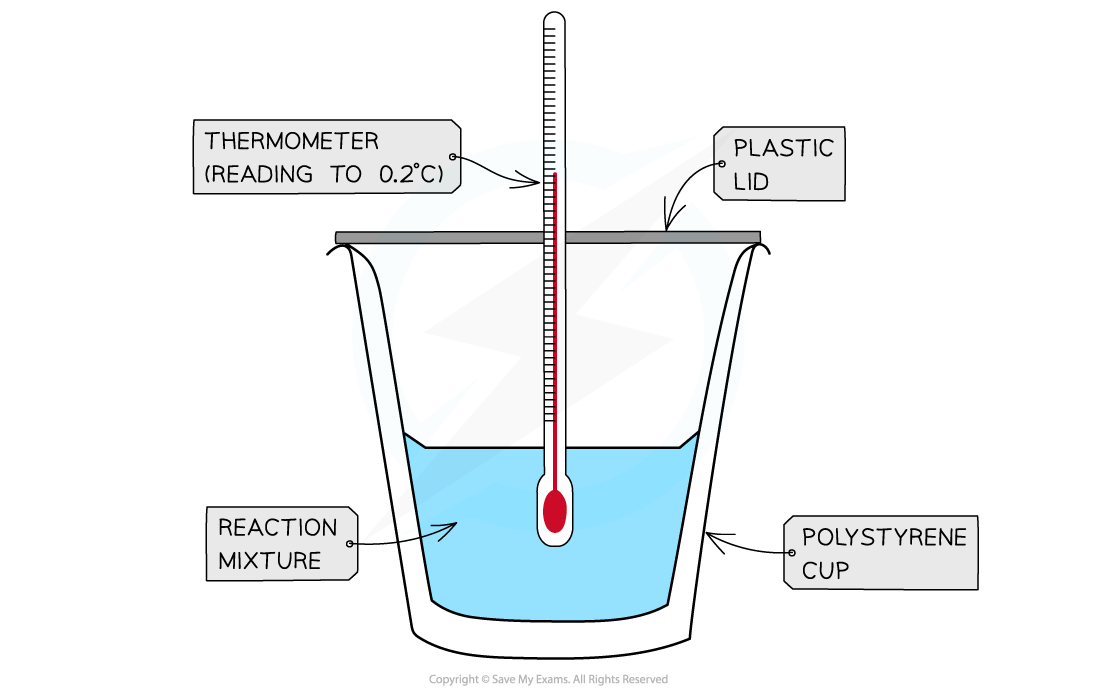
• Calorimetry can be used to find the energy change in chemical reactions.
• This is much easier to carry out in aqueous solutions
• We can take different ionic compounds in the solid phase and dissolve them in water to determine lattice enthalpy
• We must know:
  • The mass of water used
  • The mass of solid used, so recording the mass of the weighing vessel should be taken before and after

• The energy needed to increase the temperature of 1 g of a substance by 1 ^oC is called the specific heat capacity (c) of the liquid
• The specific heat capacity of water is given in the data booklet as 4.18 J g^-1 K^-1
• The energy transferred as heat can be calculated by:

Equation for calculating energy transferred in a calorimeter

• Once we know Q from the above equation, we can calculate the enthalpy change for the reaction
• Enthalpy of solution can be calculated by working out the energy change per mole of compound
• Remember, in the equation temperature change is required, i.e. the difference between the first reading and the highest / lowest reading
  • Therefore, the units for temperature do not matter as the change will still be the same in ^oC or K

Experiment 1: Addition of Ammonium Chloride and Sodium Iodide

Sample data for the reaction of ammonium chloride, NH₄Cl, and sodium iodide, NaI, with water

To calculate an experimental value for enthalpy change of the reaction, ΔH, we can follow the same basic steps:

• Step 1: Compile the relevant important information:
  • Mass of water used in experiment = 100 g
  • Temperature change = 13.8 ^oC (endothermic reaction)
  • Mass of NH₄Cl = 20.05 g
  • Molar mass of NH₄Cl = 53.50 g mol^-1

Errors in this method

• Errors in the method will lead to a difference in the data book value and the experimental value
• For this method, some of these errors are:
  • Energy transfer to the surroundings (usually loss)
    • This is the largest and most obvious error

Experiment 2: Addition of Zinc Powder to Copper(II) Sulfate

• The same calorimetry method can be used to record the temperature changes and therefore the ΔH for the reaction
• A known mass of zinc, Zn, is added to 50 cm³ of 1.00 mol dm^-3 copper(II) sulfate solution, CuSO₄ (aq), and the temperature is recorded every 30 seconds for at least 10 minutes
• A graph can be drawn from the data which shows a maximum recorded temperature of ΔT = 35.2 ^oC

Temperature change for the reaction of zinc with copper(II) sulfate

• However, we need to extrapolate the graph to when the zinc was added which gives ΔT as roughly 37 ^oC
• Important information
  • Volume of 1.00 mol dm^-3 CuSO₄ used in experiment = 50 cm³
  • Temperature change = 37 ^oC (exothermic reaction)
  • Mass of weighing bottle and Zn = 6.087 g
  • Mass of weighing bottle after emptying = 1.064 g
  • Molar mass of Zn = 65.38 g mol^-1

• For this experiment, it is slightly different as there must be a limiting reagent
• In order to identify this, we must calculate the number of moles of Zn and CuSO₄
  • Moles = mass / molar mass
    • Moles of Zn = 5.023 / 65.38
    • Moles of Zn = 0.0768 moles
  • Moles = concentration x volume (dm³)
    • Moles of CuSO₄ = 1.00 x 0.050
    • Moles of CuSO₄  = 0.050 moles
• Therefore, the CuSO₄ is the limiting reagent and should be used to calculate ΔH for the reaction
• Calculate the energy change, Q:
  • Q = mcΔT
    • Q = 50 x 4.18 x 37
    • Q = 7733 J

• Calculate ΔH:
  • ΔH = - Q / moles of CuSO₄, using -Q as it is an exothermic reaction
    • ΔH = - 7733 / 0.050
    • ΔH = -154660 J mol^-1
    • ΔH = -154.6 kJ mol^-1

Answer:

Step 1: Write an equation for the reaction occurring

HCl + NaOH → NaCl + H₂O

Step 2: Calculate the energy change for the amount of reactants in the reaction vessel (remember that the mass equals the mass of acid and alkali)

• • Q = mcΔT
    • Q = 50 x 4.18 x 13.5
    • Q = 2821.5 J

Step 3: Calculate the number of moles of HCl (remember that neutralisation has occurred)

• • Moles of HCl = concentration x volume (dm³)
    • moles of HCl = 2 x 0.025
    • moles of HCl = 0.05 moles

Step 4: Calculate ΔH, using -Q as it is an exothermic reaction

• • ΔH = - Q / moles of HCl
    • ΔH = - 2821.5 / 0.05
    • ΔH = -56430 J mol^-1
    • ΔH = -56.4 kJ mol^-1