The Interaction of Matter with Radiation (DP IB Physics: HL)

Describe what is meant by the wave function of an electron. 

An electron is confined in a finite region of length 6.8 × 10^–14 m. 

Determine the uncertainty in the momentum of the electron. 

Determine the associated de Broglie wavelength of the electron if it was accelerated into its confinement through a potential difference of 5.5 GV. 

On the axes provided, sketch the wave function Ψ of the electron described in part (b) and (c) with distance x. 

You may assume that Ψ = 0 when x = 0. 

When monochromatic light is incident on a clean metal surface, photoelectrons may be emitted through the photoelectric effect. 

Outline how Einstein's model is used to explain the photoelectric effect. 

Explain why, although the incident light is monochromatic, the kinetic energies of emitted photoelectrons vary up to some maximum.

Explain why no photoelectrons are emitted if the frequency of the incident light is less than a certain value, no matter how intense the light. 

For monochromatic light of wavelength 570 nm a stopping potential of 1.80 V is required for this particular metal surface. 

Determine the minimum energy required to emit a photoelectron from the metal surface. 

Outline the de Broglie hypothesis.

Explain why a precise knowledge of the de Broglie wavelength of an electron implies that its position cannot be measured. 

The wave function of Schrödinger's theory can be thought of as a generalisation of the de Broglie hypothesis. 

Outline the relationship between the wave function of Schrödinger's theory and the de Broglie hypothesis. 

The wave function ψ for an electron confined to length 1.0 × 10^–10 m is a standing wave as shown. 

[2]

One of the striking features of quantum theory is the ability of nature to convert matter into energy and vice versa. 

Imagine an electron moving with kinetic energy E_k on a collision course with a positron moving in the opposite direction with the same kinetic energy. Following annihilation, two photons are produced. 

Show that the wavelength λ of the two photons produced is given by the expression: 

where m_e is the mass of the electron.

Hence, show that the maximum wavelength of photons produced during this annihilation is approximately 2.4 × 10^–12 m. 

Show that the minimum wavelength of a photon that can produce an electron–positron pair is approximately 1.2 × 10^–12 m.  

Explain why the value for wavelength in part (c) is only an estimate and not an accurate result. 

Monochromatic light is incident on a metal surface and electrons are emitted instantaneously from the surface. 

Explain why:

The wavelength of light incident in part (a) is 450 nm and the work function of the metal is 2.0 × 10^–19 J. 

Determine the maximum kinetic energy of an electron emitted from the metal surface. 

The light source used in part (b) is now incident on a different metal surface. Its frequency is varied, such that the kinetic energy of emitted electrons can be recorded.  

The graph shows how the maximum kinetic energy E_K of the ejected electrons varies with the frequency of incident light. 

Use the graph to determine a value for the Planck constant h. 

Use the graph in part (c) to determine the work function of the metal.

An early form of the uncertainty principle was given by:

Later, Heisenberg refined the expression by using the "reduced Planck constant" as a way to incorporate the quantisation of angular momentum into the principle. This constant is given by:

Derive the uncertainty principle of subatomic particles in terms of:

An alpha particle is confined within a nucleus of gold-197.

Using the uncertainty principle, estimate the kinetic energy, in MeV, of the alpha particle.

It is not possible to determine the exact location of an alpha particle confined within a nucleus.

Outline how this statement is consistent with the Schrödinger model of the atom.

An alpha particle confined in a nucleus with energy E_α can be considered to be in a potential well, as shown in the diagram. The nuclear radius is equal to R.

[3]

The diagram below shows the shape of two allowed wavefunctions Ψ_A and Ψ_B for an electron confined in a one-dimensional box of length L.

On the graph, sketch a possible wavefunction for the lowest energy state of the electron.

With reference to the de Broglie hypothesis, suggest which wavefunction, ψ_A or ψ_B, corresponds to the larger electron energy.

Predict and explain which wavefunction, ψ_A or ψ_B, indicates a larger probability of finding the electron near the middle of the box.

One of the striking features of quantum theory is the ability of nature to convert matter into energy and vice versa. 

Imagine an electron moving with kinetic energy E_k on a collision course with a positron moving in the opposite direction with the same kinetic energy. Following annihilation, two photons are produced. 

Show that the wavelength λ of the two photons produced is given by the expression: 

where m_e is the mass of the electron.

Hence, show that the maximum wavelength of photons produced during this annihilation is approximately 2.4 × 10^–12 m. 

Show that the minimum wavelength of a photon that can produce an electron–positron pair is approximately 1.2 × 10^–12 m.  

Explain why the value for wavelength in part (c) is only an estimate and not an accurate result. 

Ultraviolet light is incident on a zinc plate. The zinc plate is situated within an evacuated chamber a few millimetres under a collecting plate, as shown in the diagram.

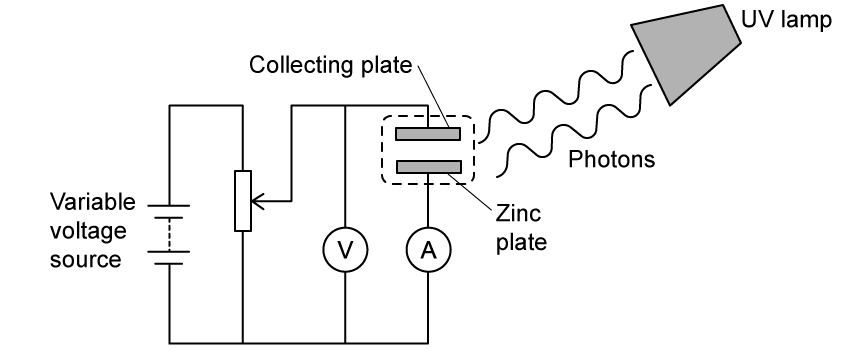
Photoelectrons are emitted from the zinc plate and move towards the positive collecting plate due to the potential difference, V, between the plates. When the potential difference, V, is varied, it is observed that the photoelectric current varies as shown on the graph. 

The experiment is repeated by using a different ultraviolet lamp which has a lower intensity and wavelength. 

The work function of zinc is 3.74 eV. 

Determine the wavelength of the ultraviolet light incident on the zinc plate.

The zinc plate has dimensions of 2.5 mm × 2.5 mm. The intensity of the light incident on the surface is 3.5 × 10⁻⁶ W m⁻². On average, one electron is emitted for every 300 photons that are incident on the surface.

Determine the initial photocurrent leaving the metal surface.

Bohr modified the Rutherford model by introducing the condition: 

. 

The total energy E_n of an electron in a stable orbit is given by:

Where 

[3]

In 1908, the physicist Friedrich Paschen first observed the photon emissions resulting from transitions from a level n to the level n = 3 of hydrogen and deduced their wavelengths were given by:

where A is a constant.

Justify this formula on the basis of the Bohr theory for hydrogen and determine an expression for the constant A.

The electron stays in the first excited state of hydrogen for a time interval of approximately ∆t = 1.0 × 10^–10 s.

Suggest, with relevant calculations, why the photons emitted in transitions from the first excited state of hydrogen to the ground state will have a small range of wavelengths.

The three lowest energy levels for an electron in a hydrogen atom are shown.

Schrodinger’s wave equation describes the boundary conditions of the three dimensions of an atom giving rise to both radial and angular allowed modes with discrete energy states. The wave equation describes the probability of finding an electron at a given point, for example, when an electron is confined in a box.